Snow load
The calculations are performed in accordance with the standard EN 1991-1-3. These procedures are included:
Snow load
The snow load on the roof is calculated using formula (5.1):
![]()
where is: | μi |
|
sk |
| |
Ce |
| |
Ct |
|
The exposure coefficient Ce is obtained according to the selected topography. The values are in accordance with the table 5.1 of EN 1991-1-3:
Topography | Coefficient Ce |
Windswept | 0.8 |
Normal | 1.0 |
Sheltered | 1.2 |
Mono-pitched roofs
The shape coefficient μ1 in accordance with figure 5.1 of EN 1991-1-3 is used for mono-pitched roofs. The value of this coefficient depends on the roof pitch:
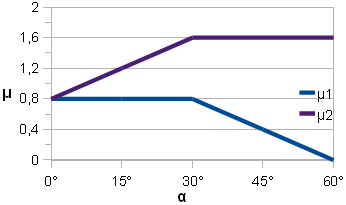 Shape coefficients μ1 and μ2
Shape coefficients μ1 and μ2
The value of the coefficient μ1 is equal to 0.8 if the sliding of the snow is prevented (snow fences etc.).
Duo-pitched roofs
The shape coefficient μ1 in accordance with figure 5.1 of EN 1991-1-3 is used for duo-pitched roofs. The figure showing the dependency of the coefficient value and the roof pitch is shown in the chapter "Mono-pitched roofs". The value of the coefficient μ1 is equal to 0.8 if the sliding of the snow is prevented (snow fences etc.). These three load cases are created for duo-pitched roof in accordance with chapter 5.3.3:
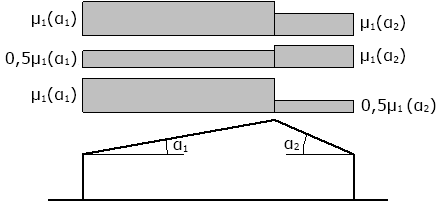 Load cases for duo-pitched roofs
Load cases for duo-pitched roofs
Multi-span roofs
The shape coefficients μ1 and μ2 in accordance with figure 5.1 of EN 1991-1-3 is used for multi-span roofs. The figure showing the dependency of the coefficients values and the roof pitch is shown in the chapter "Mono-pitched roofs". The value of the coefficient μ1 is equal to 0.8 if the sliding of the snow is prevented (snow fences etc.). These load cases for undrifted and drifted snow are created in accordance with chapter 5.4:
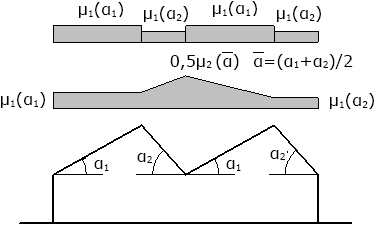 Load cases for multi-span roofs
Load cases for multi-span roofs
The snow load shape coefficient μ1 for an exceptional snow drift that will be used for valleys of multi-span roofs is given in chapter B.2 of EN 1991-1-3 and in following diagram:
 Shape coefficient and drift lengths for exceptional snow drifts
Shape coefficient and drift lengths for exceptional snow drifts
Shape coefficient μ1 is determined as the least value of:
The drift lengths are determined as:
![]()
Cylindrical roofs
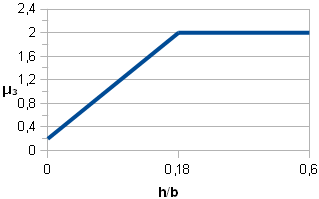
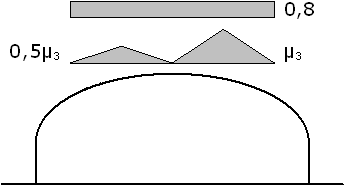
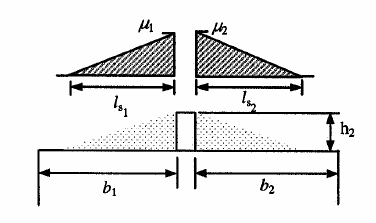
The shape coefficient μ3 in accordance with figure 5.3.5(1) of EN 1991-1-3 is used for cylindrical roofs. Its value is depending on ratio h/b, where h is the height of cylindrical roof and b is the roof span. The coefficient values are shown in the following figure:
 Coefficient μ3
Coefficient μ3
The snow load is considered in the parts where the roof pitch is smaller than 60° according to the chapter 5.3.5. Following load cases are considered:
 Load cases for cylindrical roofs
Load cases for cylindrical roofs
Following load case based on the standards CSN/STN 73 0035 is considered additionally for Czech and Slovak nation annexes :
 Drifted snow in accordance with Czech and Slovak national annex
Drifted snow in accordance with Czech and Slovak national annex
This load case is considered under these circumstances:
- This scheme will be considered for all cylindrical roofs with ratio h/b greater than 1/8.
- This scheme will be considered for all cylindrical roofs in snow areas IV and V.
Roofs abutting and close to taller construction works
The shape coefficients for these roofs are calculated according to the chapter 5.3.6 of EN 1991-1-3. These load cases are considered:
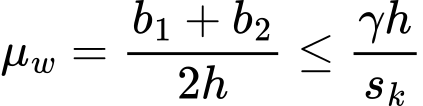 Load cases for abutting roofs
Load cases for abutting roofs
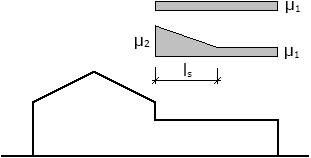
The shape coefficients μ1 and μ2 are calculated using following formulas:
![]()
Where is: | μs |
|
μw |
|
The value of the coefficient μs is equal to 0 for α ≤ 15°. The following formula is used for α > 15°:
![]()
Where is: | μ |
|
bs |
| |
ls |
|
The coefficient μw is calculated using following formula:
![]()
Where is: | b1 |
|
b1 |
| |
h |
| |
γ |
|
The drift length ls is calculated using formula
![]()
where is: | h |
|
The drift length ls is limited by the interval <5m;15m>. The shape coefficient is calculated using lineat interpolation between μ1 and μ2 for buildings where ls is greater than span of lower roof.
The snow load shape coefficients μ1 and μ2 for exceptional snow drifts that should be used for roofs abutting a taller construction work are given in chapter B.3 of EN 1991-1-3 and in table B.1 and following picture:
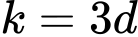 Shape coefficients and drift lengths for exceptional snow drifts
Shape coefficients and drift lengths for exceptional snow drifts
The drift length ls is the least value of 5h, b1 or 15 m.
Drifting at projections and obstructions
The following loading figure is created in accordance with the chapter 6.2 of EN 1991-1-3 for the roofs with obstructions:
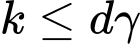 Loading figure for obstructions
Loading figure for obstructions
The coefficients μ1 and μ2 are calculated using formula (6.1):
![]()
where is: | γ |
|
h |
| |
sk |
|
The snow load shape coefficients μ1 and μ2 for exceptional snow drifts that should be used for roofs where drifting occurs at projections and obstructions, other than parapets, are given in chapter B4(2) of EN 1991-1-3.
 Shape coefficients for exceptional snow drifts for roofs where drifting occurs on flat roof
Shape coefficients for exceptional snow drifts for roofs where drifting occurs on flat roof
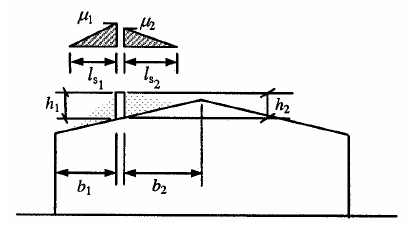 Shape coefficients for exceptional snow drifts for roofs where drifting occurs on pitched or curved roof
Shape coefficients for exceptional snow drifts for roofs where drifting occurs on pitched or curved roof
The shape coefficient is determined as the least value of:
![]()
The drift length lsi is taken as the least value of 5h or bi, where i = 1 or 2 and h ≤ 1 m.
Snow overhanging the edge of a roof
Snow overhanging the edge of a roof is calculated in accordance with chapter 6.3. Following formula is used:
![]()
where is: | k |
|
s |
| |
γ |
|
The coefficient k is calculated using formula:
![]()
But following expression has to be fulfilled:
![]()
where is: | d |
|
Snow load on snowguards and other obstacles
Snow load on snowguards and other obstacles is calculated according to the chapter 6.4 of EN 1991-1-3. Following formula is used:
![]()
where is: | s |
|
b |
| |
α |
|